Unveiling the Enigma: Gibbs Entropic Paradox And the Intricacies of Separation Processes

The field of thermodynamics has long been a source of fascination and intrigue for scientists and researchers alike. Within the realm of this captivating discipline, one particular topic that stands out is the Gibbs Entropic Paradox and its implications for separation processes. In this article, we will delve into the depths of this enigmatic paradox, uncover its underlying principles, and explore the challenges faced in separation processes.

Understanding the Gibbs Entropic Paradox

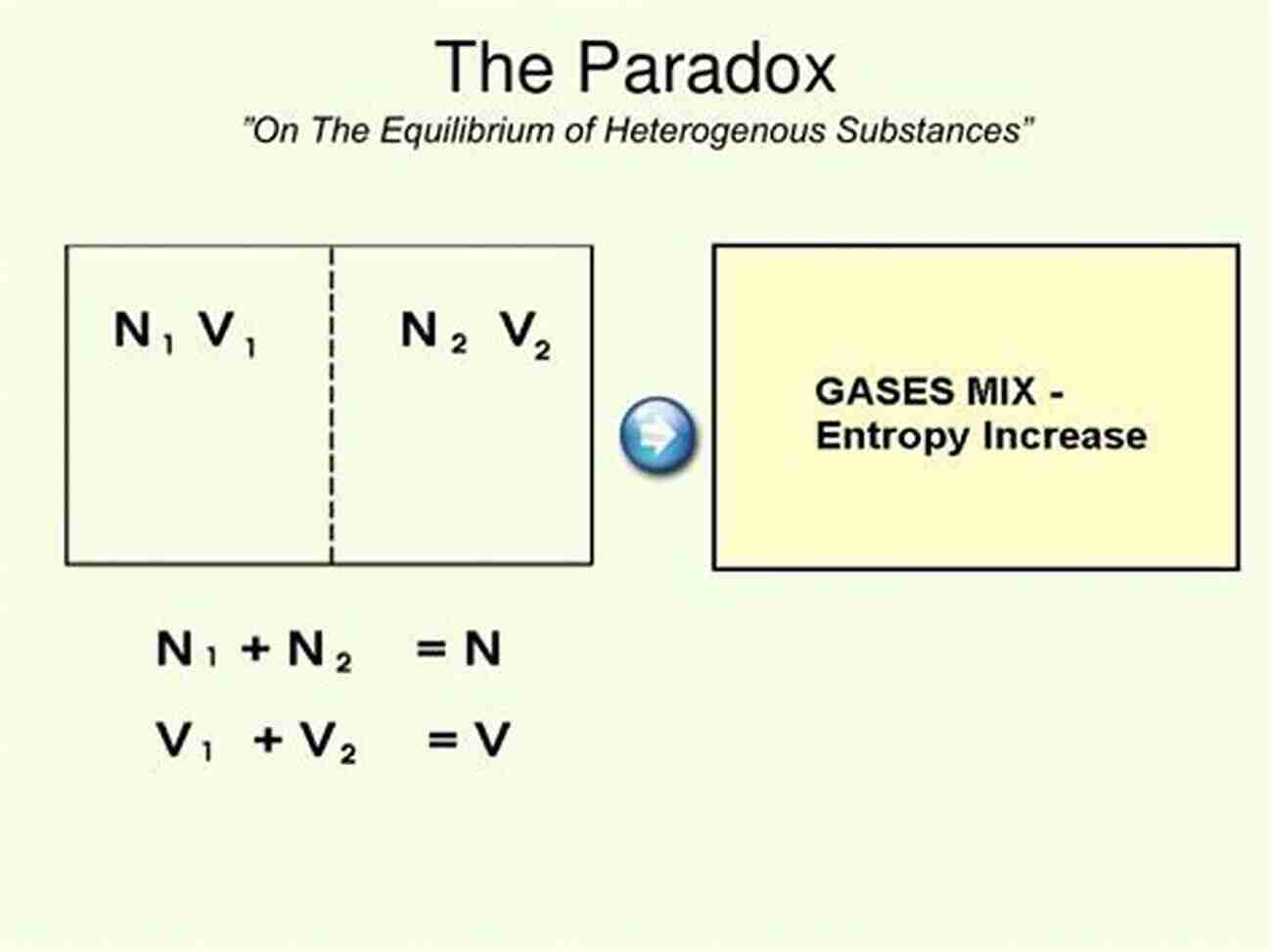
The Gibbs Entropic Paradox, named after the distinguished physicist Josiah Willard Gibbs, revolves around the concept of mixing two different substances with precision and the consequences in terms of thermodynamic entropy. According to the second law of thermodynamics, the entropy of an isolated system tends to increase over time, leading to a more disordered state.

However, the paradox arises when considering the mixing of two substances, like different gases, in a closed system. Intuitively, one would expect the entropy of the system to increase as the two gases mix and disperse evenly. Nonetheless, Gibbs demonstrated that in certain circumstances, this mixing process results in a decreased overall entropy.

Gibbs' Entropic Paradox and Problems of Separation Processes

by Eric Rosenberg(1st Edition, Kindle Edition)

5 out of 5

Language	:	English
File size	:	15685 KB
Text-to-Speech	:	Enabled
Screen Reader	:	Supported
Enhanced typesetting	:	Enabled
Print length	:	189 pages

FREE

Gibbs' work on the paradox revealed that the entropy decrease is counterbalanced by an enthalpy increase, a measure of the total heat content of a system. This compensation allows for a decrease in entropy while conserving energy within the system, apparent violation of the second law of thermodynamics at first glance.

The Implications for Separation Processes

The Gibbs Entropic Paradox poses significant challenges for separation processes that rely on principles of thermodynamics. Separation processes involve the extraction of specific components from a mixture, such as purifying water or separating different gases.

Traditionally, separation processes rely on creating an equilibrium between two phases, such as a liquid phase and a vapor phase, and exploiting the differences in chemical properties between the components. The aim is to achieve a maximum separation efficiency while minimizing energy consumption.

However, the Gibbs Entropic Paradox presents hurdles to this conventional separation approach. In certain scenarios, the energy required to overcome the decrease in entropy during the separation process may outweigh the benefits gained. This often leads to inefficiencies and challenges in achieving the desired levels of separation.

Overcoming the Challenges

Researchers and engineers in the field of separation processes face the task of finding innovative solutions to overcome the challenges posed by the Gibbs Entropic Paradox. One approach involves incorporating advanced techniques, such as membrane separation, where membranes with selective permeability are utilized to separate components based on size and molecular properties.

Another promising avenue is the development of complex algorithms and computational modeling to optimize separation processes. By leveraging the power of simulations, scientists can explore various scenarios, analyze system behavior, and identify potential improvements in efficiency and effectiveness.

The Future of Separation Processes

The study of the Gibbs Entropic Paradox has opened up new avenues for research in separation processes. As scientists continue to enhance their understanding of this paradox and develop innovative techniques, we can expect significant advancements in the field.

These advancements will prove invaluable in diverse industries such as chemical engineering, pharmaceuticals, and environmental sciences. By maximizing separation efficiency and minimizing energy consumption, the repercussions will extend beyond scientific communities, playing a vital role in shaping our future sustainable processes.

The Gibbs Entropic Paradox stands as an enigma within the realm of thermodynamics, challenging our understanding of entropy and energy conservation. Through ongoing research, scientists aim to unlock its intricacies and harness its potential to overcome the challenges faced in separation processes.

As we continue to unravel the mysteries that govern the laws of nature, the Gibbs Entropic Paradox serves as a humbling reminder of the complexities that lie before us. By embracing these challenges, we pave the way for groundbreaking advancements that will shape our future.

Gibbs' Entropic Paradox and Problems of Separation Processes

by Eric Rosenberg(1st Edition, Kindle Edition)

5 out of 5

Language	:	English
File size	:	15685 KB
Text-to-Speech	:	Enabled
Screen Reader	:	Supported
Enhanced typesetting	:	Enabled
Print length	:	189 pages

FREE

Gibbs' Entropic Paradox and Problems of Separation Processes reviews the so-called Gibb’s Paradox observed during the mixing of two systems. During the last 150 years, many physicists and specialists in thermodynamics, statistical and quantum mechanics been engaged in the solution of the Gibbs paradox. Many books and journal articles have written on this topic, but a widely accepted answer is still lacking. In this book, the author reviews and analyzes all this data. Based on findings, the book formulates a different approach to this paradox and substantiates it on the basis of physical and statistical principles.

The book clearly shows that entropy consists of two parts, static and dynamic. Up to now, entropy has been connected only with the process dynamics. However, the Gibbs paradox is caused by the change in the static component of entropy. Finally, the book includes examples of separation processes and how to optimize them in various fields, including biology, cosmology, crystallography and the social sciences.

• Provides a precise definition of entropy and allows the formulation of criteria for optimization of separation processes
• Explains the role of entropy in many processes, facilitating an in-depth analysis and understanding of complicated systems and processes
• Provides solutions to scientific and applied problems in various scientific disciplines related to separation processes
• Elucidates entropy’s role in many separation systems